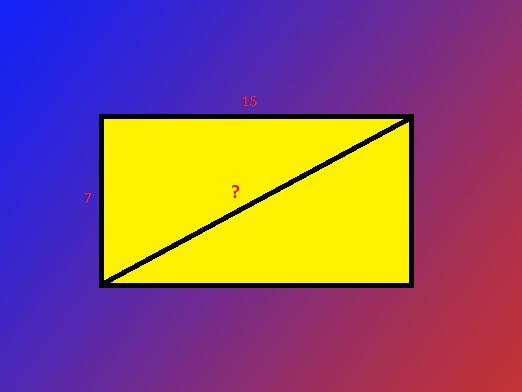
How to find the length of the third side of the triangle?

The problem of solving triangles (this is the name of such problems) is handled by a special section of geometry - trigonometry.
The length of the two sides of the triangle
Find the length of the third side of the trianglerectangular suggested the ancient mathematician Pythagoras known to everyone. The basis is a rectangular triangle, that is, one in which one of the angles is equal to 90 degrees. The adjacent sides to this corner are always referred to as the legs, respectively, the third, the largest side, is called the "hypotenuse". Pythagoras' theorem is as follows: "the square of the length of the hypotenuse is equal to the sum of the squares of the lengths of the legs."
To solve such a problem, the value of the length of oneWe designate the leg as X (X), and the other Y (yoke), the length of the hypotenuse can be designated as Z (z). Now let's write the formula for calculating the length of the hypotenuse: Z squared = X squared + Y squared. Proceeding from such a formula, we finally get the value of the square of the length of the hypotenuse. Hence, to obtain the value of the length of the hypotenuse, it is still necessary to extract the square root of the resulting sum of the lengths of the legs.
Previously, we considered the ideal option, whenit is necessary to determine the length of the hypotenuse. If, in the problem, the length of one of the legs is unknown, then, based on the above theorem, we can derive the derived formula. The square of the length of one of the legs is equal to the value obtained by subtracting the square of the length of the other leg from the square of the length of the hypotenuse: X squared = Z squared - Y squared. Well, the last action is necessarily the extraction of the square root of the obtained value.
For example, take the simple values of lengthcuvettes: 2 and 3 centimeters. By simple mathematical operations, we get Z squared = 4 + 9 = 13. This means that Z is approximately 3.6 centimeters. If we exclude the squaring of the values, it turns out that Z = 2 + 3 = 5 centimeters, which does not correspond to the truth.
By the length of the two sides and by the value of the angle between them
It is possible to find the length of the third side of the triangle,using the cosine theorem. This geometric theorem is as follows: the square of one of the sides of the triangle is equal to the value obtained by subtracting the doubled product of the length of the known sides and the cosine of the angle that lies between them, from the sum of the squared lengths of the known sides.
In mathematical form such a formula looks likeas follows: Z squared = X² + Y²-2 * X * Y * cosC. Here, X, Y, Z denote the length of all sides of the triangle, and C is the value in degrees of the angle that is located between the known sides.
For example, we use a triangle, knownwhose sides are equal to 2 and 4 centimeters, and the angle between them is 60 degrees. We use the above formula and get: Z squared = 4 + 16-2 * 2 * 4 * cos60 = 20-8 = 12. The length of the unknown side is 3.46 centimeters.