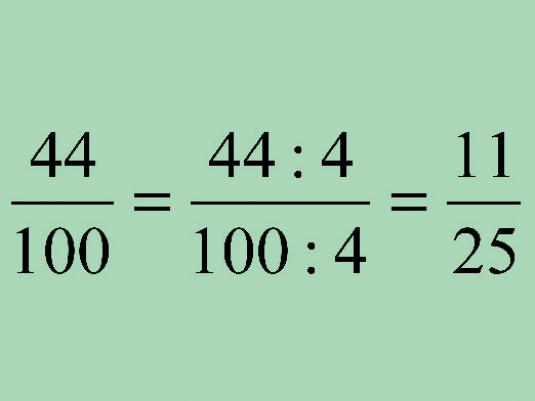How to add fractions?

To understand how to add fractions, you must first of all know the classification of fractions.
Types of fractions
- Ordinary fractions
- Correct fractions
- Wrong fractions
- Mixed numbers
Fold decimals
How to add decimals? In the addition of decimals, the record is used with a "column", so that the corresponding bits are placed one above the other without a shift. In this case, the commas are exactly one above the other.
Example:
2.45; 5.76
4.65; 5.73
Weak decimals in the "column", as well as natural numbers, despite the presence of commas. In the answer, a comma is placed under commas in both fractions.
If the decimals to be collated contain differentthe number of digits after the decimal point, then to fractions, where fewer decimal places are required to assign the missing number of zeros in order to equalize the number of decimal places in the fractions.
Find the sum of fractions: 0.534 + 22.5
Equalize the number of decimal places in these fractions. We add two zeros to the right to the decimal fraction of 22.5.
- 0.534 + 22.500 =
- 00,534
- 22,500
- 23,034
Fold ordinary fractions
How to add ordinary fractions? Let two fractions be given to you:
- 2/7 and 2/3
We find the common denominator for these fractions. It will be equal to the product of their denominators.
We reduce fractions to the common denominator. We multiply the numerator of the first fraction with the denominator of the second fraction, and the numerator of the second fraction with the denominator of the first fraction, so the denominators of both fractions will be 21. We get the following:
- 6/21 and 14/21
We reduce these fractions, then we obtain a fraction with the common denominator - we reduce the numerators of the reduced fractions. The denominator will remain the same. That is, we get:
- 6 + 14 = 20
- 20 and will be the result of addition of 2 and 2
- It turns out 20/21
Mixed fractions
A fraction that is written in the form of an integer nearthe correct fraction, is called a mixed fraction and it is understood as the sum of the assigned number and fraction. Each rational number can be written as a mixed fraction. The opposite part of the mixed fraction - contains only the numerator and denominator.
Example:
2 2 6 2 8
2 - = 2 + - = - + - = -;
3 3 3 3 3
Thus, to perform any action, the mixed fraction can be brought into the form of a normal fraction for the solution in an appropriate way.